Nonlinear elasticity and viscoelasticity |
Nonlinear elasticity is the subject that deals with materials that are capable of large, reversible deformations. Of specific importance are deformations of polymeric composites, elastomers and soft tissues. Of course, in reality, no deformations are perfectly reversible - this is an approximation and we are required to incorporate lossy effects such as viscoelasticity. Nonlinear elasticity and viscoelasticity are very active areas of research in the group, both in terms of the mathematical apparatus and the application of the theory to problems in the applied sciences.
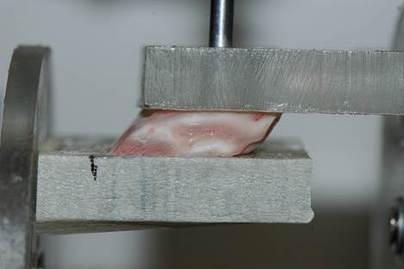
The picture to the right shows porcine brain matter under simple shear, illustrating the large deformations possible in soft tissues. Thanks to our good friend Michel Destrade (NUI Galway) for this nice image taken from his paper entitled "Extreme softness of brain matter in simple shear" which was published in the International Journal of Non-linear Mechanics.
Within the MWM group, we are interested in the fundamental theory underlying nonlinear viscoelasticity. In 2014 we reformulated the quasilinear theory of viscoelasticity originally proposed by Fung. Although this theory does have its limitations, it does have merits and some of the recent criticisms in the literature are, in fact, unfounded, as we showed in our 2014 Proceedings A paper. We also then extended this to the context of transversely isotropic materials. Our work on biological tissues has tried to ascertain the correct form of strain energy functions for the hyperelastic behaviour of tendons and ligaments. We have also developed homogenization techniques in order to predict the associated effective nonlinear viscoelastic behaviour. This incorporates strain dependent relaxation via "fibril recruitment". Finally we have done significant work on the ability of nonlinear elasticity to provide a tuning mechanism to waves propagation. In particular we showed that certain nonlinear elastic materials have an invariance property which means that they have the ability to act as natural cloaks under pre-stress. See our metamaterials page and waves in pre-stressed media page for further information.
Journal of The Royal Society Interface, 14(128), 20160867.
The picture to the right shows porcine brain matter under simple shear, illustrating the large deformations possible in soft tissues. Thanks to our good friend Michel Destrade (NUI Galway) for this nice image taken from his paper entitled "Extreme softness of brain matter in simple shear" which was published in the International Journal of Non-linear Mechanics.
Within the MWM group, we are interested in the fundamental theory underlying nonlinear viscoelasticity. In 2014 we reformulated the quasilinear theory of viscoelasticity originally proposed by Fung. Although this theory does have its limitations, it does have merits and some of the recent criticisms in the literature are, in fact, unfounded, as we showed in our 2014 Proceedings A paper. We also then extended this to the context of transversely isotropic materials. Our work on biological tissues has tried to ascertain the correct form of strain energy functions for the hyperelastic behaviour of tendons and ligaments. We have also developed homogenization techniques in order to predict the associated effective nonlinear viscoelastic behaviour. This incorporates strain dependent relaxation via "fibril recruitment". Finally we have done significant work on the ability of nonlinear elasticity to provide a tuning mechanism to waves propagation. In particular we showed that certain nonlinear elastic materials have an invariance property which means that they have the ability to act as natural cloaks under pre-stress. See our metamaterials page and waves in pre-stressed media page for further information.
- Parnell, W.J. and De Pascalis, R. (2019) (open access)
"Soft metamaterials with dynamic functionality tuned by pre-deformation"
Phil. Trans. A, 377, 2144. - De Pascalis, R., Parnell, W.J., Abrahams, I.D., Shearer, T., Daly, D.M. and Grundy, D. (2018) (open access)
"The inflation of viscoelastic balloons and hollow viscera"
Proc. R. Soc. A. 474, 20180102 - Balbi, V., Shearer, T. and Parnell, W.J. (2018) (open access)
"A modified formulation of quasi-linear viscoelasticity for transversely isotropic materials under finite deformation"
Proc. R. Soc. A. 474, 20180231 - Zhang, P. and Parnell, W.J. (2017)
"Band gap formation and tunability in stretchable serpentine interconnects"
ASME J. Applied Mechanics. In press. - Gower, A.L., Shearer, T. and Ciarletta, P. (2017) (open access)
"A new restriction for initially stressed elastic solids"
Quarterly Journal of Mechanics and Applied Mathematics 70(4), 455-478. - Paoletti, P., Jones, G. W., and Mahadevan, L. (2017).
Journal of The Royal Society Interface, 14(128), 20160867.
- Barnwell, E.G., Parnell, W.J. and Abrahams, I.D. (2016) (open access)
"Antiplane elastic wave propagation in pre-stressed periodic structures; tuning, band-gap switching and invariance"
Wave Motion 63, 98-110. - Shearer, T., Parnell, W.J. and Abrahams, I.D. (2015) (open access)
"Antiplane wave scattering from a cylindrical cavity in pre-stressed nonlinear elastic media"
Proc. R. Soc. A. 471, 20150450 - Shearer, T. (2015) (open access)
"A new strain energy function for modelling ligaments and tendons whose fascicles have a helical arrangement of fibrils"
J. Biomech. 48, 3017-3025 - De Pascalis, R., Abrahams, I.D. and Parnell, W.J. (2015) (open access)
"Simple shear of a compressible viscoelastic material"
Int. J. Eng. Science 88, 64-72. - Shearer, T. (2015) (open access)
"A new strain energy function for the hyperelastic modelling of ligaments and tendons based on fascicle microstructure"
J. Biomech. 48, 290-297 - Gilchrist, M., Murphy, J.G., Parnell, W.J. and Pierrat, B. (2014)
"Modelling the slight compressibility of anisotropic soft tissue"
Int. J. Solids Structures. 51, 3857-3865 - De Pascalis, R., Abrahams, I.D. and Parnell, W.J. (2014) (open access)
"On nonlinear viscoelastic deformations - a reappraisal of Fung's quasilinear viscoelastic model"
Proc. Roy. Soc. A 470 (2166) - De Pascalis, R., Parnell, W.J. and Abrahams, I.D. (2013)
"Predicting the nonlinear pressure-volume curve of an elastic microsphere composite"
J. Mech. Physics Solids 61, 1106-1123
Our collaborators
|
From the UK
Artur Gower, University of Sheffield
|
From around the world
|