One of the things researchers often wrestle with is creating clear and succinct explanations of their research for the non-specialist.  It can be extremely helpful to work with others; such as illustrators, teachers and performers, to eliminate jargon and create meaningful outputs that showcase the research.

When we wanted to make a short video about the design of metamaterials for noise reduction devices, we turned to illustrator and comedian John Cooper.

John has worked on projects for the University of Manchester before, creating work for the University’s School Governor Initiative and for the Children’s University of Manchester.  His humorous style lends clarity and informality to a topic.

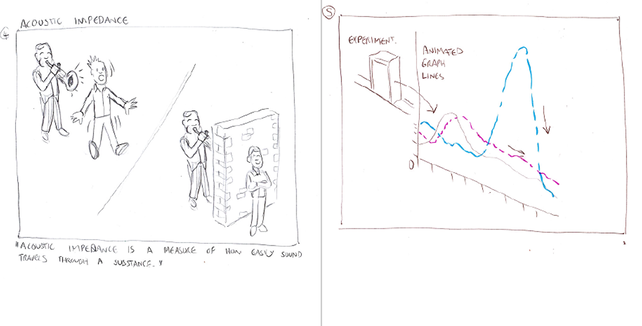
We started from a blog post about the piece of research in question.  John used it to sketch out an initial storyboard proposal featuring noisy geese!  We then put together an initial script, from which John created a slideshow storyboard.

This project was completed during lockdown, so all our discussions were carried out over email or video conferencing.  Keeping the length of the script to a minimum was challenging, but after several iterations we arrived at the final version, which John narrates.

Here’s what John had to say, ‘I really enjoyed this project. The work the department does is fascinating, and it was an exciting challenge in generating visuals to complement their work on noise reduction.  It's good to learn new things while being creative.’
​
Here’s the finished product.  Watch out for those geese!

We have prepared and tested a series of polymer-filled syntactic foams.  Foams with a high proportion of polymer microspheres showed excellent recovery and damage resistance in compression tests.

The first syntactic foam was developed in 1955 by the Bakelite Company of New York, and hailed as “A plastic foam, which promises to cut the partial cost of boat and airplane construction as much as 50%” [1].  It offered strength, insulation and tuneable properties in a lightweight material.

Syntactic foams are materials made up of hollow microspheres (commonly of glass, ceramic or plastic) held in a polymer matrix.  The strength and buoyancy of syntactic foams has led to their widespread use in marine applications, their sound absorbing properties find uses in acoustic applications, and they have even been used in World Cup footballs due to their low density and elastic recovery [2,3]. 

Glass vs plastic

The mechanical behaviour of materials can be defined in terms of stress (the force applied to the material per unit area) and strain (the deformation in the material in response to stress).

Viscoelastic materials combine the behaviours of viscous fluids and elastic solids when deformed. A plot of stress vs strain shows hysteresis, where the unloading (reverse) curve follows a different path to the loading (forward) curve, because some energy is lost to the system as heat:

Our study
​
In this study we manufactured and tested polyurethane (PU) syntactic foams containing two grades of polymer microsphere.  The foams contained a 2%, 10% or 40% volume of microspheres.

The syntactic foams were tested up to medium (25% and 50%) strain alongside unfilled polyurethane.  Samples were compressed and unloaded five times and the response measured.

The foams showed viscoelastic behaviour, with hysteresis in the stress-strain curves.  Samples with a low volume of microspheres showed similar behaviour to unfilled PU, but increased proportions of microspheres led to some different results.  The 10% and 40% samples exhibited stress softening, where a smaller force is needed to achieve the same deformation in successive loadings.  This may be due to the buckled microspheres not fully recovering between load cycles.

After 1 week, the samples were re-tested.  They showed the same behaviour and little or no change in thickness, indicating that they had fully recovered from the previous testing.

​Microscopy reveals cracks in the polyurethane in the damaged samples.  The microspheres mitigate the damage by presenting a barrier to crack propagation.  This is the opposite result to the case with glass and ceramic microspheres, where foams with higher volume fractions are less resistant to damage, due to the brittle nature of the microspheres.

These results indicate that polymer-filled syntactic foams containing higher volume fractions of microspheres can show good elastic recovery and significant damage resistance.  They may provide an excellent alternative to glass and ceramic containing syntactic foams for applications that require low density materials with high resistance to damage under strain.
 
Read the full article here: doi.org/10.1016/j.compositesb.2020.107764

[1] Plastic Foam Developed for Boats and Planes, The Science News-Letter, 1955, 67(14),  213
[2] N. Gupta, S.E. Zeltmann, V.C. Shunmugasamy, D. Pinisetty Applications of polymer matrix syntactic foams, JOM, 2014, 66(2), 245-254
[3] For explanation of the anatomy of the 2014 Adidas Brazuca World Cup football: www.livescience.com/46299-microscopic-analysis-brazuca-world-cup-ball.html

We have developed a new model for the complex viscoelastic behaviour of tendons, which fits experimental data, and allows the behaviour of tendons under stress to be predicted accurately.

The USA reports 33 million musculoskeletal injuries per year, of which about half involve damage to soft tissues including tendons and ligaments (1).  Tendon repair is often slow, and incomplete (2).   An understanding of the mechanical behaviour of tendons is essential to the development of effective surgical reconstruction and prosthetic tissues.

Tendons are made up of fascicles: bundles of collagen fibrils embedded in a matrix containing water,  proteins such as elastin, and other substances.  Fibrils are up to 60 times thinner than spider silk, and crimped (folded in a concertina fashion).  ​Tendons connect bone to muscle, while ligaments connect bone to bone.

Viscoelastic materials
Tendons exhibit non-linear viscoelastic behaviour.  Viscoelastic materials combine the behaviours of both viscous fluids and elastic solids when deformed. Their mechanical behaviour is described in terms of stress (the force applied to the material per unit area) and strain (the deformation in the material in response to stress).

In a viscoelastic material:

• A plot of stress vs strain shows hysteresis, where the state of a system depends on its history: when stress or strain is increased and then decreased, the outward and return graphs follow different curves:

​Our m​odel
Our model builds on existing models of tendon behaviour to account for fibril creep.  We model the individual behaviour of the fibrils and matrix as linear viscoelastic, but because we allow for a distribution of different lengths of fibril in a fascicle, we can account for the non-linearity that arises in the large-scale behaviour.
 
Strain is a measure of the change in length of the fascicle compared to its undeformed length. We consider the fascicle to contain multiple crimped fibrils, each with a critical length, where it is fully straightened and becomes taut.  The fibrils only begin to take up strain when they are taut.  This is illustrated in the clip below: 

The red threads represent fibrils.  As we stretch the fascicle the fibrils begin to straighten. The shortest fibrils are the first to take up strain.  By the end of the clip the bottom fibril has reached its critical length and begins to take up strain.  Stiffness increases as the stretch in the fascicle increases, and more fibrils reach their critical strain length.
 
When the stress is released, the fibrils begin to slacken.  Because of the viscoelastic memory effect, the critical strain at which a fibril straightens will change with each sequential load.
 
We can illustrate the model in two tests: relaxation test and cycle test.

​Relaxation test
In the relaxation test the fascicle is rapidly stretched (strained), then held constant for a time, and this is repeated in a stepwise fashion, illustrated by the top graph (below, left).  The middle graph shows the corresponding strain in a fibril which reaches its critical length and begins to take up strain at time tA.  The bottom graph shows the fibril stress, and the relaxation effect is seen.

​Cycle test
In the cycle test the fascicle is gradually stretched then unloaded.  This is repeated twice (top graph, below, right).  The graphs below it show the associated strain (middle) and stress (bottom) in a fibril.
 
On first unloading, the stress relaxation and creep effects are seen. As a result of the first cycle, the critical strain length for the second loading is longer than the first for fibrils that have taken up strain in the first cycle. For fibrils that do not straighten fully in the first cycle, the critical length will be unchanged. 

​Time-evolution
The overall response of the fascicle depends on the relative proportions of matrix and fibrils, and the distribution of fibril lengths.  Crucially, in our model, accounting for fibril creep allows us to predict how the fibril length distribution will evolve over time.  The animations below show how the length distribution changes during the cycle test.  The animation on the left does not account for fibril creep, while the one on the right does.  

​This element of our model allows a close fit to experimental data and provides a better understanding and more accurate prediction of tendon behaviour.

Read the full paper here: https://doi.org/10.1115/1.4045662

(1) F. Wu, M. Nerlich and D. Docheva, Tendon Injuries, Basic Science and New Repair Proposals, EFORT Open Reviews, 2017, 2, 332-342 10.1302/2058-5241.2.160075
​(2) R. James, G. Kesturu, G. Balian and A. Chhabra, Tendon: Biology, Biomechanics, Repair, Growth Factors, and Evolving Treatment Options, The Journal of Hand Surgery, 2008, 33, 102-112 doi.org/10.1016/j.jhsa.2007.09.007

We have modelled, designed and printed a metamaterial with a structure that reduces the effective speed of sound in air by half, opening up the potential for space-saving noise cancellation devices.

Noise pollution can reduce our quality of life, and even our life expectancy.  A 2014 report from the European Environment Agency estimates that 10,000 premature deaths and 43,000 hospital admissions for coronary heart disease and stroke can be attributed to noise exposure in Europe each year. (1)  The urgent need for efficient noise cancellation devices is clear.

Noise cancellation devices often work on the principle of destructive interference, where sound waves are combined so that they cancel each other out.
​
Here’s how it works:

One tool that may be employed to reduce noise from engines, fans and other devices is a Quarter Wavelength Resonator (QWR).  This is a side branch in a duct that redirects sound waves so that they interfere destructively.  The length of the QWR is ¼ of the wavelength of the noise frequency to be attenuated. 

​The animation below shows how a QWR functions:

Part of the sound wave is diverted into the QWR, reflects off the end wall and then recombines with the undiverted wave.  The diverted wave has travelled an additional ½ wavelength, so the waves now re-combine destructively, cancelling the noise. 
​
There are limitations to this approach: the size of the resonator depends on the wavelength of the noise we want to reduce.  Low frequency noises have long wavelengths, so the size of the resonator required soon becomes impractically large.

We used a mathematical mapping process called Transformation Acoustics to design and 3D print a metamaterial that has the potential to overcome the size limitations of QWRs for reducing low frequency noise.

Metamaterials are special materials with properties that are not found in conventional materials.  Unlike conventional materials, the properties of metamaterials are defined by their structures rather than by their chemical make-up.  Acoustic metamaterials have geometries that allow the manipulation of sound waves in ways not previously achievable.

Our metamaterial has arrays of elliptical cylinders which stretch the apparent space inside the resonator, with the result that the effective speed of sound is reduced by half.  Crucially, it does this while maintaining a close match to the impedance of sound in air across a range of frequencies. 

​One of the most rewarding experiences of my PhD is being able to attend to conferences and the opportunity to introduce myself and present my research to a wide audience of professionals. After two and a half years of PhD studies, I have had the chance to visit some great places within the UK and Europe, and now Australia! KOZWaves is a biennial conference focused on the study wave science and is always held somewhere within Australia and New Zealand. I first heard of it in my PhD first year and thought how amazing it would be to get to know a new community and give a talk at this event. Two years later I was very happy to receive the news that my talk: "Wave Propagation in Thermo-Visco-Elastic Continua" had been accepted to KOZWaves 2020 which was held at the University of Melbourne from the 17-19th of February.
 
The conference exceeded my expectations with great speakers talking from gravitational waves to water waves as well as light, sound and vibrations (to name just a few!) They showcased - the well known fact of - how waves describe so many physical phenomena of the world that surrounds us.

​The next step of the journey was a visit to the University of Adelaide in South Australia which is an 80 minute flight from Melbourne's Tullamarine Airport. As a keen surfer myself, before taking the flight I paid a visit to URBNSURF, a new facility for surfing artificial waves (again waves!) in a big pool right by the airport which was super fun!

I spent three fantastic days at the University of Adelaide visiting Dr. Luke Bennetts. I gave a talk to the Mechanics group on "Modelling Thermo-Viscous Damping in Continua". 

Finally, I visited Dr. Stuart Hawkins at Macquarie University in Sydney and some of his colleagues including Dr. Elena Vynogradova and her PhD student Martin Sagradian. I gave an hour long talk similar to the one I presented in Adelaide and had some interesting conversations. Stuart showed me some very impressive numerical computations via the use of the T-matrix approach for multiple scattering problems.